Continuum Mechanics in Mechanical Engineering
The research of the Continuum Mechanics group is focused on the cross-scale continuum mechanical modeling of applied materials, such as metallic polycrystals and fiber-reinforced polymers. The focus is on problems of material modeling as well as the effective homogenization of material properties. In material modeling, the framework of linear and nonlinear continuum thermomechanics is used. Numerical methods (FEM, FFT, DeepMaterialNetworks) as well as statistical methods (Mean Field Theories, Maximum Entropy Method Approaches) are used for the scale-bridging of the material behavior. Another focus is the description of microstructures by microstructural parameters such as fiber orientation tensors and tensorial texture coefficients and their incorporation into continuum mechanical models. Microstructure evolution is modeled and simulated for textures in metallic materials in forming processes and for fiber reinforced polymers in injection molding. In addition, characterization methods (biaxial testing, dynamic mechanical analysis, dilatometry, thermography) are used to identify material models.
Nonlinear continuum mechanics and materials theory |
| The aim of continuum mechanics (or continuum thermomechanics when temperature is taken into account) is to model the (thermo)mechanical behaviour of materials and structures on scales where the atomic structure of materials need not be taken into account. The main areas of continuum mechanics are kinematics, balance equations and material theory, in particular the implications of the second law of thermodynamics in the formulation of material equations. |
Modelling of heterogeneous media |
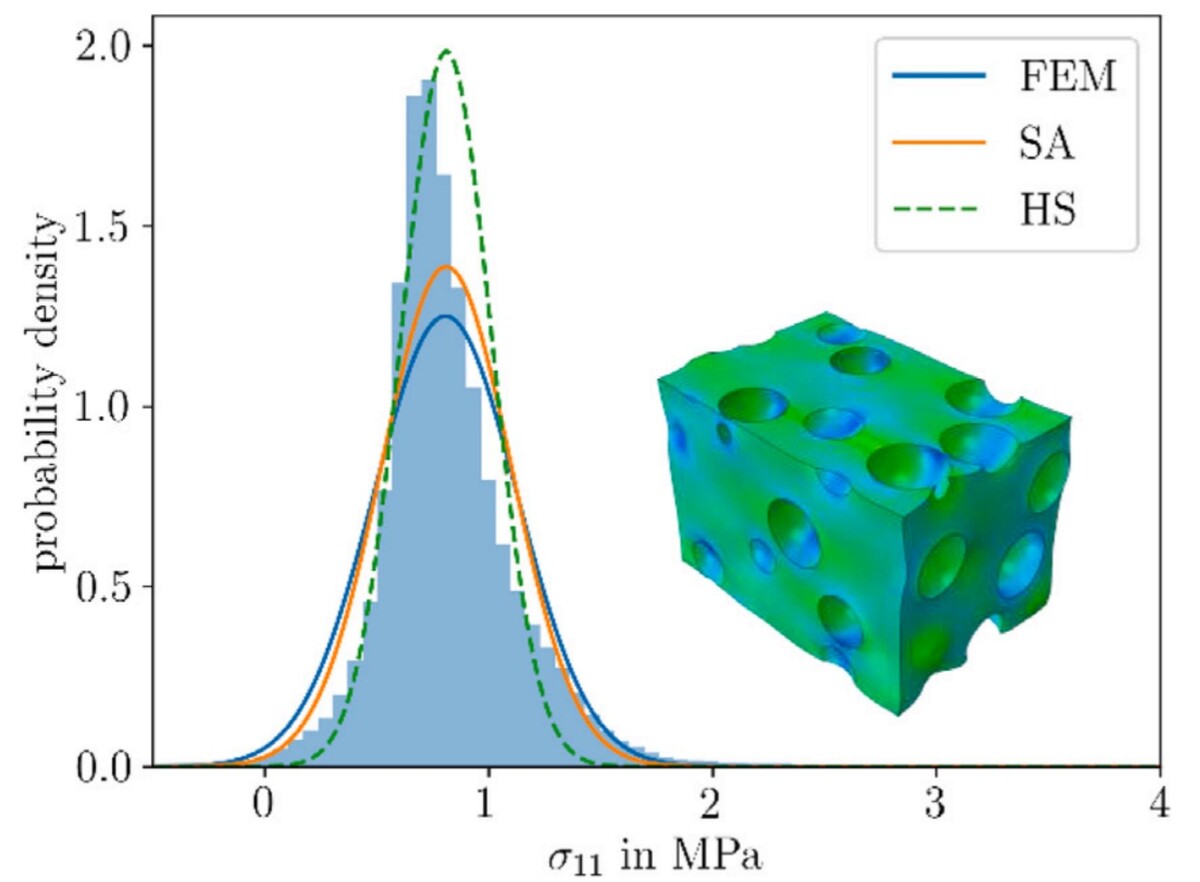
| Micromechanical methods derive the macroscopic behaviour of materials by homogenising material properties at the micro level and microstructural information e.g. Mean Field Theory or Maximum Entropy Method. An example is the prediction of the directional properties of fibre reinforced polymer materials in lightweight construction. These properties are determined, for example, by the distribution of fibre orientation and fibre length, which can be determined using tomographic methods. |
Micromechanical full-field simulation of applied materials using FEM and FFT |
| In the case of non-linear material behaviour, the homogenisation of material properties often has to be carried out purely numerically. The Finite Element Method and Fast Fourier Transformation can be used for this purpose. Examples of such problems are the non-linear thermo-viscoelastic behaviour of fibre reinforced polymers or the creep behaviour of directionally solidified high temperature materials. |
Thermomechanical modelling of metals and polymers |
| Applied materials show a change in material response under varying mechanical and thermal loads. The material behaviour can be e.g. elastic, plastic, viscoplastic. Numerical simulations require material models that reliably describe the material behaviour under a variety of loading conditions. The development of such models is based on a combination of continuum mechanical and mathematical concepts. |
Model Identification |
| Simulations with continuum mechanical models require that the material parameters and functions of the models used are known. These parameters and functions depend on the microstructure induced by the manufacturing process. Thermomechanical properties are measured experimentally in the Chair's Thermomechanical Laboratory and used to identify the models (Biaxial Testing, Dynamic Mechanical Analysis, Dilatometry, Thermography). |
Testing Technology
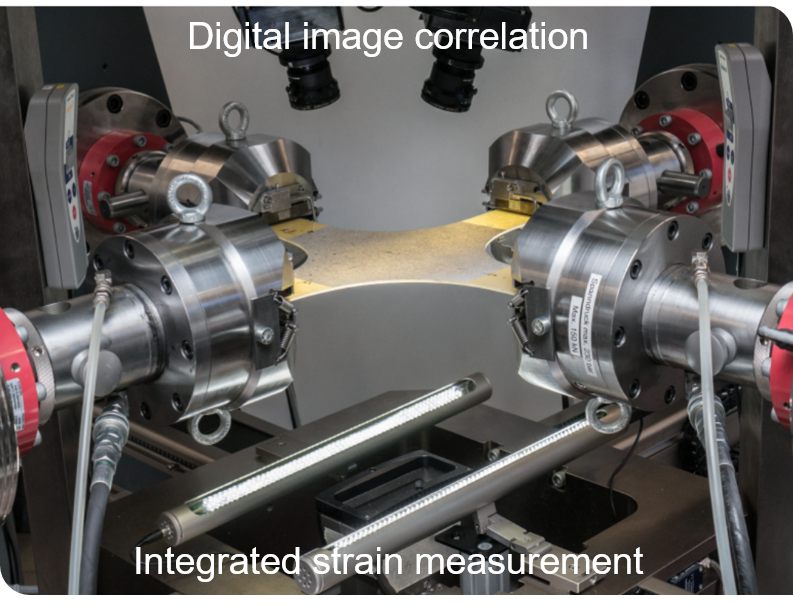
| Electromechanical biaxial testing device with 4 separately controlled testing axes (Zwick) |
| Optical strain measurement by means of DIC (Aramis 3D 4M (GOM)) (biaxial |
| Dynamic mechanical thermal analysis (DMA), EPLEXOR® 500 N (GABO) |
| Dilatometer, DIL 402 Expedis® Select (Netzsch) |
| Laserflash, LFA 467 HyperFlash® (Netzsch) |
| Drying and heating chamber (Binder) |
| High-precision weighing device (Kern) |
| Desiccator (Sicco) |